Rollin' In Their Graves
The number of people alive today are over 1/10th of the people who ever lived.
I'm not sure where I first heard that curious statistic, but it is exactly the kind of thing I like to repeat. Is it true? I mean, I know there are a lot of people in China and India, as well as a bunch of people in the US, Indonesia, Brazil, Pakistan, Nigeria etc, but to compare that to all the people? Ever? In all 6,000 30,000 200,000 years of human existence on Earth? Assuming the zombie apocalypse, would we really just need to destroy 9 zombies each? That sounds quite doable! (Side note, it feels really good to write a statistical blog and start a sentence with, "Assuming the zombie apocalypse".)
Oh, The Humanity
One problem we run into is the definition of what it means to have "all of humanity". It means there was a beginning. We separated from our closest surviving primate ancestors (chimps) a few million years ago, but were still mingling and struggling against other hominids until much more recently. Our small populations at the time make the annual human contribution quite tiny, but the sheer variation in start date magnifies the importance of the question. One solution is to use our pinch point. About 100,000 years ago the population of early humans was reduced to possibly as few as 2,000 individuals. After this, we were clearly our own species, so I will use 100,000 BCE as our start date and 2,000 as our starting population. Other data I pulled off the US Census website where they have nice global estimates for 10,000 BCE through 1950 at which point they have precise annual estimates.
First let's model their data. I put date in Column A (using negatives for BCE) and population (in millions) in Column B. The initial plot looks kind of exponential.
You can see how big a role artificial fertilizers were. Since their introduction, the doubling period of global population growth has been 50-ish years. This is obviously not sustainable, but we will come back to that later.
But we don't want to know how many people there were in any given year. We want to know how many people there were total. To do this we will count the one thing everyone has had... a birth. Even if they only lived a few minutes or are still alive today, at some point they were born. (I hadn't thought about it until now but a significant portion of the zombie apocalypse will be children. It is sad, but it does improve our chances.) The number of births in a given year can be calculated by the crude birth rate (CBR) which is total births per 1,000 people per year. This number can be found for modern eras and estimated for ancient ones. It ranges from 50-ish in cultures with high infant mortality, limited women's rights, and limited contraception to 10-ish in cultures with the opposite. Both extremes put pressure on society (to care for the young or the elderly, respectively), but if you are the leader of a culture you would probably prefer the latter.
Anyways, put CBR in Column C and we'll estimate that pre-1950 it is about 37.2 (our earliest data point). If we average the population over our different time spans and multiply by years and CBR we get approximate number of people born during that time span. We make that equation by typing the [in brackets] portion. Move everything down so data starts in Row 4 and we have room for calculations.
(Cell D4) [=0.002] Million People Initially
(Cell D5) [=(C5/1000)*AVERAGE(B4:B5)*(A5-A4)]
Complete the column. Column E will be how many people are born up to that point. And Column F will be the ratio of current population to people ever.
(Cell E4) [=sum(D$4:D4)]
(Cell F4) [=A4/E4*100]
Complete the columns. Cool! We really are about 1/8th of the population ever. Here are some representative data points.
Making the CBR of nomadic hunter gatherer people's 20 or 50 (which are both defensible positions without much real data) only changes this by about 3%. Interestingly, back when I heard the statistic, it might have been 1/10th of the population. The fraction of "All People" who are currently alive has been steadily growing.
Making the CBR of nomadic hunter gatherer people's 20 or 50 (which are both defensible positions without much real data) only changes this by about 3%. Interestingly, back when I heard the statistic, it might have been 1/10th of the population. The fraction of "All People" who are currently alive has been steadily growing.
But what will happen in the future?
One Hundred... Billion... Dollars. What? People? That Doesn't Make sense.
To answer that, lets first have a little aside. If you thought the growth of the twentieth century was scary, the following graph should be downright terrifying.
Keeping with the 50-year doubling trend would mean that in 200 years we could be looking at 100 billion people. Even discounting the Earth's ability to sustain such a population, each person would only have a third of an acre of Earth's land-surface area. Unless we started building underground. This is obviously silly, right?
It May Be A Growing Problem
At what point will global growth slow? Lets look forward to futuristic date of... 1970. Yeah, this totally threw me for a loop too. I was taking the second derivative of population with respect to time, you know, like you do, and bamb! It is glaring. Here is an even easier way to look at it. Find the average annual growth rate for each period:
(Cell G5) [=EXP(LN(B5/B4)/(A5-A4))-1]
Complete the column and you see that for most of human history there has been low or no growth in population followed by furious activity in the last thousand (and especially hundred) years.
Lets zoom in.
Correlation is not causation, but it certainly looks like artificial fertilizer helped increase the growth rate. As for the decline, it could be many things. China instituted its one-child policy, many economies slowed down, but importantly, lots of countries modernized their view about contraception and women's rights. If we extrapolate the rate of growth, we eventually get down to zero. At that point our population would stabilize at right around 10 billion people.
So that slowing is good news for the planet (also confirmed by the US Census and UN independent estimations), but bad news for our odds in the zombie apocalypse. Even worse is that we start losing our edge even before the population stabilizes.
Undead Reckoning
Lets take a minute to think about what we really want to know and what we already know. We know there is a ratio of "Current Population" to "All People Ever". We know this ratio changes. We want to know when that ratio is going to stop changing in the positive direction, and start going down. In math or physics, we would call this "finding the maximum of a curve" and would accomplish this by defining the derivative of the ratio with respect to time and finding where it goes to zero. That is where it stops changing. If you dislike reading the maths, this might be a good time to skip to the graph. Basically we are doing a related rates problem:
We already have the rate of current population growth, but to bring this forward, I first need to tell you how I made the extrapolations. Start by taking a derivative of population growth. This is just the change in population growth over a change in time.
(Cell H6) [=(G6-G5)/(A6-A5)]
Complete the column, and you have the increase or decrease in population growth per-year. This data is often very noisy, but recently it has been remarkably stable, with a value of around -0.03%. The negative means that the rate of population growth is decreasing each year. To extend it past 2013, I calculate the moving average of the previous 40 years:
(Cell H107) [=AVERAGE(H67:H106)]
When you complete the column (and add a hundred years to column A) you can extrapolate the growth rate:
(Cell G107) [=G106+H107]
And you can increase (or decrease) your future population and total of "All People Ever":
(Cell B107) [=B106*G107]
(Complete all other rows)
(Add in estimates of CBR for future years)
Now we are back to the graph I showed previously, so let's look at the growth rate for "All People Ever":
(Cell I5) [=EXP(LN(E5/E4)/(A5-A4))-1]
Plotting these two on the same graph demonstrates that the "Current Population" rate passes over the "All People Ever" rate well before the "Current Population" rate reaches zero (and our population stops growing).
So our best chances are in the year 2059 when 15.21% of All People Ever will currently be alive. This may seem counter intuitive since "Current Population" will still be growing, but one rationalization is that while "All People Ever" is just based on births, "Current Population" is based on births and deaths, meaning that "All People Ever" will start growing faster than "Current Population". (And theoretically, integrating the area under the Current Population curve between the "maximum ratio" date and the "population stabilizes" date will yield a number of people that will die after the best zombie apocalypse date but before current population decreases.)
Boneheaded Oversight
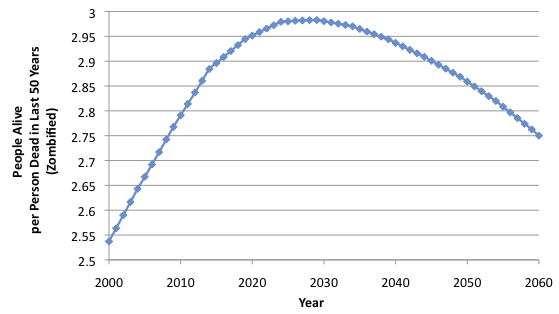
Of course, as my wife mentioned to me when looking over my first draft, we shouldn't really be afraid of people who are dead for such a long time that they are just bones. Those aren't real zombies. Let's limit it to just people who might still be creepy looking. What is the ratio of people alive today to people who died in the last 50 years?
(Cell J44:J206) = Crude Death Rate (CDR)
(Cell K44) [=(J44/1000)*AVERAGE(B43:B44)*(A43-A44)]
(Cell L93) [=A93/SUM(K44:K93)]
Complete the columns and graph.
Looking at it this way our odds are much better. There are currently 2.86 people alive for each person dead in the last 50 years. On the other hand, our advantage goes away even sooner, due to the stabilization of our CDR. Here's hoping that the zombie apocalypse is right around the corner?
-----










No comments:
Post a Comment